Decays Continuously With a Half life of 3 Years
Exponential Decay Formula
Before knowing the exponential decay formula, first, let us recall what is meant by an exponential decay. In exponential decay, a quantity slowly decreases in the beginning and then decreases rapidly. We use the exponential decay formula to find population decay (depreciation) and we can also use the exponential decay formula to find half-life (the amount of time for the population to become half of its size). Let us learn more about the exponential decay formula along with the solved examples
What are Exponential Decay Formulas?
The Exponential decay formula helps in finding the rapid decrease over a period of time i.e. the exponential decrease. The exponential decay formula is used to find the population decay, half-life, radioactivity decay, etc. The general form is f(x) = a (1 - r)x.
Where
a = initial amount
1-r = decay factor
x= time period
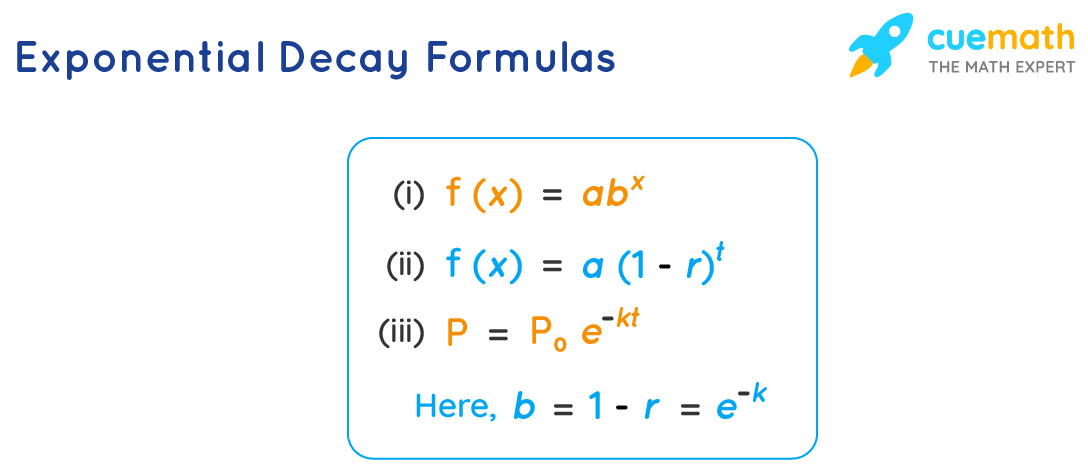
Exponential Decay Formula
The quantity decreases slowly after which the rate of change and the rate of growth decreases over a period of time rapidly. This decrease in growth is calculated by using the exponential decay formula. The exponential decay formula can be in one of the following forms:
f(x) = abx
f(x) = a (1 - r)x
P = P\(_0\) e- k t
Where,
- a (or) P\(_0\) = Initial amount
- b = decay factor
- r = Rate of decay (for exponential decay)
- x (or) t = time intervals (time can be in years, days, (or) months, whatever you are using should be consistent throughout the problem).
- k = constant of proportionality
- e- Euler's constant
Note: In exponential decay, always 0 < b < 1.Here, b = 1 - r ≈ e- k.

Break down tough concepts through simple visuals.
Math will no longer be a tough subject, especially when you understand the concepts through visualizations with Cuemath.
Book a Free Trial Class
Examples Using Exponential Decay Formulas
Example 1: Chris bought a new car for $20,000. The value of the car decreases exponentially (depreciates) at a rate of 8% per year. Then what is the value of the car after 5 years? Solve this by using exponential formulas and round your answer to the nearest two decimals.
Solution:
The initial value of the car is, P = $20,000.
The rate of decay is, r = 8% = 0.08.
The time is t = 5 years.
Using the exponential decay formula:
A = P (1 - r)t
A = 20000 (1 - 0.08)5= 13181.63
Therefore, the value of the car after 5 years = $13,181.63.
Example 2: Jane bought a new house for $350,000. The value of the house decreases exponentially (depreciates) at a rate of 5% per year. Then what is the value of the house after 2 years? Solve this by using exponential formulas and round your answer to the nearest two decimals.
Solution:
The initial value of the house = $3,50,000
The rate of decay, r = 5% = 0.05
The time, t = 2 years
Using the exponential decay formula,
A = P (1 - r)t
A = 350000 (1 - 0.05)2
A = 315,875
Therefore, the value of the house after 2 years = $315,875
Example 3: The half-life of carbon-14 is 5,730 years. Find the exponential decay model of carbon-14. Solve it by using the exponential decay formula and round the proportionality constant to 4 decimals.
Solution:
Using the given data, we can say that carbon-14 is decaying and hence we use the formula of exponential decay.
P = P\(_0\) e- k t
Here, P\(_0\) = initial amount of carbon
It is given that the half-life of carbon-14 is 5,730 years. It means
P = P\(_0\) / 2 = Half of the initial amount of carbon when t = 5, 730.
Substitute all these values in the formula of exponential decay:
P\(_0\) / 2 =P\(_0\) e- k (5730)
Dividing both sides by P\(_0\),
0.5 = e- k (5730)
Taking "ln" on both sides,
ln 0.5 = -5730k
Dividing both sides by -5730,
k = ln 0.5 / (-5730) ≈ 1.2097
Thus, the exponential decay model of carbon-14 is P = P\(_0\) e- 1.2097k
FAQs on Exponential Decay Formulas
What is Exponential Decay Formula?
The quantity decreases slowly at regular intervals by a fixed percent. This decrease in growth is calculated by using the exponential decay formula. The general form is f(x) = a (1 - r)x,
where
- a = Initial amount
- r = Rate of decay
- x = time interval
The exponential decay formula is used to find the population decay, half-life, radioactive decay, etc.
What is the Formula For Finding the Exponential Decay?
The exponential decay formula helps in finding the rapid decrease over a period of time i.e. the exponential decrease. This decrease in growth is calculated by using the exponential decay formula. The exponential decay formula can be in one of the following forms:
f(x) = a (1 - r)x(general form)
P = P\(_0\) e- k t (for continuous exponential decay)
where,
- a (or) P\(_0\) = Initial amount
- b = decay factor
- r = Rate of decay
- x (or) t = time intervals
- k = constant of proportionality
- e- Euler's constant
How Do You Calculate The Exponential Decay?
The exponential formula is y = abx. Here b is the decay factor. The decay is calculated as (1-r), where r = decay rate. Now y is the decay function. y = a (1-r)x.
How Do You Find The Decay Rate of An Exponential Function?
The exponential decay formula is f(x) = a bx , where b is the decay factor. The decay rate in the exponential decay function is expressed as a decimal. The decay rate is given in percentage. We convert it into a decimal by just dropping off % and dividing it by 100. Then find the decay factor b = 1-r. For example, if the decay rate is 12%, then decay rate of the exponential function is 0.12 and the decay factor b= 1- 0.12 = 0.88
Source: https://www.cuemath.com/exponential-decay-formula/
0 Response to "Decays Continuously With a Half life of 3 Years"
Enregistrer un commentaire